
Assignment #11: Polar Equations
by
Laura Singletary

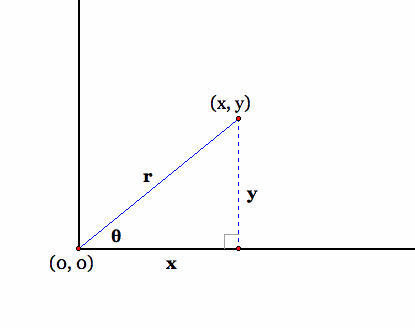
(Inspired by Wolfram Mathematics)
Ok, now let us begin our exploration!

To begin our exploration when a and b are equal and k is an integer, consider the basic case and let a = b = k =1, this provides us with a graph known as a cardioid ( or a 1-leaf rose):

What happens when a = b = -1 and k =1?

What about when a = b = 2 and k =1? Notice the cardioid passes through (0, 2) and (0, -2) since a = b = 2, rather than (0, 1) and (0, -1) when a = b =1. Notice as k = 1, this is still a 1-leaf rose.

When a = b = k = 2 ? Looks like an infinity symbol or a 2-leaf rose.

What about when a = b = 3 and k =2? Could we conjecture that it is still a 2-leaf rose as k = 2?

What about when a = b = k = 3? Might this be a 3-leaf rose?

What about a=b=3 and k=4? A 4-leaf rose?

While exploring what happens when a and b are equal and k is an integer, it seems that we have discovered that when k is an integer it determines how many leaves form the n-leaf rose. Similarly, a and b seem to affect the length of the petals of the rose. Before attempting the second portion of the exploration, I wondered what might happen if k was not an integral value, and so I allowed a=b=3 and k=4.5. Notice what happens. We have a rose with 4.5 petals.

Because graphing calculator allows for changing parameters so easy, I wondered what happens for large values of k. Look at what happens when k=303. Why did this happen? Isn't it beautiful?

Part 2: What happens when a and b are not equal and k is equal to 1? Consider when a = 3 and b = 5 (Notice a < b):

Consider when a = 3 and b = 7 (Notice a < b):
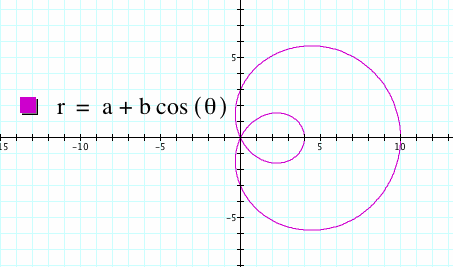
Consider when a = 7 and b = 4 (Notice a > b):

Consider when a = 7 and b = 5 (Notice a >b):

So what is happening when we vary the values for a and b? (I got my trusty old college Calculus book out for this one to name these curves appropriately.) There were obvious differences in the curves when comparing curves with a > b and the curves created when a < b. First, these curves produced by our polar equation when a does not equal b are called "Limacons". When a < b, we have a limacon with an inner loop. When a >b, we have a dimpled limacon.
For fun: I wondered what would happen if we varied a, b, and k.
Consider when a=3, b=5, k=5.

Consider when a = 3, b = 7, k = 5

Consider when a = 7, b =3, k = 5

Consider when a = 7, b = 5, k = 5

So what is happening when we vary the values for a and b and k? It seems as is we have merged the ideas about how k determines the number of petals on the rose along with how varying the values of a and b (depending on if a >b or if a <b) determines if we have "dimples" or "inner loops". Interesting? I thought so!
Part 3: What happens if we replace cos ( ) with sin ( )?
How changing the trigonometric fuction affects the graph is easily seen when comparing the following two equations (the polar equations for a circle, we will allow a = 1):

The orientation of the curve changes when you replace cos ( ) with sin ( ). Explore this concept with some of the other polar equations we have looked at in this investigation. Why do you think the orientation changes? What do we know about trigonometry that would help us understand why the orientation of these curves is different? Have fun exploring polar equations!